SPREADING THE WORD: THE 6DB DECAY PER DOUBLE DISTANCE CHALLENGE.

When a sound source moves closer, the sound gets louder and vice versa - everybody knows that. It’s one of the main challenges to cope with in the design of sound reinforcement systems, so engineers soon learn about the ‘6dB per double distance’ rule that says it all. The rule is based on a simple physical concept: the distribution of acoustic energy.
First things first… Because of their construction, loudspeaker driver units by definition have a certain dispersion behaviour: the radiated acoustic energy - or sound intensity - decreases the more you go off-axis. Most loudspeaker dispersion specifications are published as -6dB ‘coverage’ points for mid-frequencies, broadening for lower frequencies, narrowing for higher frequencies. The on-axis focus is sometimes given as the ‘directivity index’ of the speaker, ‘Q’.
So, given that we measure or listen far enough away from the speaker so the speaker acts as a point source, the radiated sound energy is distributed over a surface which is dependent on the distance from the speaker and its dispersion behaviour. Imagine blowing up a balloon: the more air put into the balloon, the further away the balloon’s skin is from the centre and the larger the surface. The coverage properties of the balloon determine the shape: a Q of 1 produces a perfect ball, a very high Q may produce the sausage shape that balloon artists use to make balloon dogs. It’s the same with loudspeakers: Q=1 produces a pure omnidirectional distribution, where higher Q’s focus energy on-axis.
Shaped by the loudspeaker’s dispersion properties, the sound energy is spread out over an expanding surface as the sound waves move away from the speaker - similar to blowing up the balloon. If we measure (or listen to) the sound intensity (I) - which is the sound energy per area measured in watts per square metre - at a certain distance (r), by detecting the sound using just a small spot on the surface (e.g. the surface of the measurement microphone, or the effective surface of our ear canal) this gives us the related sound pressure (p), measured in Pascal, or Newton (force) per square metre. This is the acoustic property we actually perceive and measure.
If we measure or listen to the same speaker at twice the distance, we know the surface that the sound intensity is distributed over will have grown by the square of the increase, because their relationship is that of a ball’s surface:
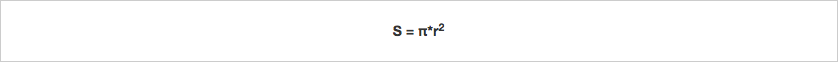
where

This immediately implies that the sound intensity measured over a fixed area will decrease with the square of the distance ratio. As we’re discussing power, if we represent the sound intensity ratio as sound intensity level (SIL) in decibels (dB), we get:

The same goes for Sound Pressure Level (SPL) - see the previous tutorial blog: remember that we measure SPL as indication of the power relation. As a calculation: sound pressure (p) relates to the square root of sound intensity (I), but by using the factor 20 we get the same dB value for the field property SPL as with the power property SIL.
Now it becomes clear that, for any distance from the speaker, if we double the distance we get an SIL and SPL decay of 20*log( 2r / r ) = 20*log(2)... which is approximately 6dB.
Now we know this, we can address the most important challenge in designing ‘Public Address’ sound reinforcement systems: to make sure everybody in the audience hears roughly the same sound level.
First, we need to set an acceptable difference of SPL between the listeners who are closest to and furthest from the loudspeaker. Let’s use an arbitrary difference of 6dB. If we then place the loudspeaker at ear height at one metre from the closest listener (sitting in the centre of the first row), the furthest listener (at the centre of the last row) can be only one metre further away: two times 6dB equals two times the distance. For a very small pub this one metre ‘spread’ would serve just a few listeners; for larger audiences we need to think of something else.
The solution is to increase the distance to the closest listener and decrease the distance ratio to the furthest listener. The constraint is the stage depth - to prevent feedback, the speakers have to be in front of the vocalists, and there’s always a limit to how far we can keep the first row of listeners away from the stage edge. Mounting the speakers on speaker stands to place them higher - or, in installed systems, simply suspending the speakers from the ceiling - helps a great deal. This increases the distance to the closest listener, and at the same time lowers the distance ratio of the closest and furthest listener. In the example of the very small pub with a ceiling height of three metres, the spread increases from one metre to three metres - already suitable for live music in a small pub.
For larger audiences, we may need to apply a distributed system and add delayed speakers or use speaker systems with different energy distribution properties, such as column speakers or line arrays. These are topics for future episodes of this micro tutorial blog.
For now, understanding the 6dB per double distance challenge gives us a useful design tool to cope with design challenges for small to medium size venues - which form the majority of uses for sound reinforcement systems.